Anne B. Abell and David A. Lange

Department of Civil Engineering

University of Illinois

Urbana, IL 81801 USA

The texture of fracture surfaces is key evidence regarding the behavior of materials. Deflection, microcracking and bridging are toughening mechanisms in fracture of brittle matrices that affect surface roughness. This study combines mechanical testing with two optical techniques that map the geometry of fracture surfaces. The confocal laser microscope and a video density technique provide elevation data for the mesoscale of mortar and macroscale of concrete. Recently, the surface maps have been used to establish a link between surface roughness and fracture parameters, as well as to investigate the fractal nature of these materials. The tortuosity of the crack can be used in a micromechanical model to predict the increase in toughness due to deflection caused by aggregates. The deflection angle of the crack in the direction of propagation as well as perpendicular to it is used to determine the local strain energy release rate for the mixed mode condition at the crack tip. The analysis is performed for two sets of mortar bars to investigate the influence of the aggregate. One set varies the maximum aggregate size of the sand distribution, while the other has mono-sized aggregate of varying diameters. The influence of crack deflection will be compared to tested fracture parameters.

Characterization of fracture surfaces is an area of investigation that provides understanding about the relationship of geometry and microstructure to the mechanical behavior of mortar and concrete. Mechanisms involved in fracture such as microcracking, aggregate deflection, bridging and roughness induced closure are indicated by physical evidence or artifacts during or after the separation of the material with a macrocrack. Images of the surface obtained from scanning electron microscopes (SEM), optical and confocal laser microscopes, and video capture provide qualitative information about the fracture. In addition, the confocal microscope and a video density technique obtain quantitative data of the surface with a topographic map. This data can be analyzed to determine the roughness and fractal nature of the surfaces, and can be used as input for a micromechanical model that predicts the local fracture toughness increase from the crack deflection. Fracture toughness values determined from mechanical testing, resulting in the fractured surfaces, can be compared to the surface parameters.

Confocal microscopy has been used to conveniently obtain elevation data for cement paste and mortar fracture surfaces [1]. This technique assembles a series of optical sections taken at different focal planes into a digital image where every x,y coordinate (pixel) value is assigned a z-level from the section with the brightest value at that location. Video capture has also been applied to concrete fracture surfaces to obtain island boundaries by Issa, et al. [2]. The technique obtained images of the surface which was covered with varying depths of a colored, non-staining fluid. The boundaries were also determined from thresholding of gray values which represented the elevation height of interest. This elevation density technique has recently been adapted to obtain the surface topology of paste and mortar surfaces.

The topological information provided by both methods can be used to determine the surface area from the geometric construction of triangular planes defined by the x-y-z coordinates of each pixel. From this area, a surface roughness number (RN) can be found. The coordinates can also be used to determine the plane or facet orientation, profile or surface fractal dimension, and the crack profile deflection angles.

A mechanics of materials model which predicts the fracture toughness increase due to crack deflection around second phase particles in a matrix was proposed by Faber and Evans [3]. Based on the reduction in local stress intensity at the crack tip when it is deflected or when the crack plane is bowed, the model predicted the average strain energy release rate due to particle morphology, aspect ratio, spacing and volume fraction of the second phase. The topographic maps from the image techniques mentioned provide the actual crack tortuosity so that the deflection (tilt) and bowing (twist) angles can be input directly into the model to estimate the fracture toughness increase from that of a flat crack through the plain matrix.

The effect of aggregate size and gradation on the material properties and fracture surface geometry of mortars was of interest in this investigation. Mortar as a simpler composite material (two phases) than concrete, can be modeled directly with the mechanics of materials model. The materials were tested for fracture toughness and the resulting surfaces were imaged.

The materials tested were a mortar of ordinary Portland cement (OPC) with a w/c ratio of 0.45 and 1:3 ratio by weight of cement to sand, and an OPC paste of w/c ratio of 0.45. Two sample sets of mortars were designed. The fine aggregate was graded with standard sieve sizes of 9.5mm, No. 4, 8, 16, 30, 50 and 100 and the distribution in comparison to ASTM C33 limits is presented in Table 1. The maximum aggregate set consisted of four designs which eliminated the particles collected on sieves above the sizes of No. 4, 8, 16 and 30. The surfaces for the maximum aggregate set are shown in Figure 1a. The mono-sized aggregate set consisted of three designs which used the particles collected on the sieve sizes No. 8, 16 and 30, and the surfaces are shown in Figure 1b. Silica sand of ASTM grades 20/30, 50/70 and ASTM C190 were used for the gradation from sieves No. 30 through 50 to reduce the incidence of aggregate fracture. River sand was used for all other sieve sizes.

Table 1. Fine Aggregate Base Grading

a)

b)

Figure 1. Fracture surfaces of the sample sets a) mono-sized (sieve) aggregate

samples and paste, b) maximum aggregate samples and paste

The mortars and paste were cast into bars of size 38.1 mm (1.5 in.) ´ 25.4 mm (1 in.) ´ 177.8 mm (7 in.) with a notch of 12.7 mm (0.5 in.) in height at mid-span. Four bars were cast of each mix. The bars were moist cured for 28 days prior to testing.

Three-point bend tests were performed on the specimens following the Two-Parameter Fracture Model method [4]. The loading span was 152.4 mm (6 in.), and typical load-crack mouth opening displacement (CMOD) curves for the maximum aggregate set is shown in Figure 2. The critical stress intensity factor, K_Ic, and Young's Modulus determined from the tests are shown in Table 2.

Figure 2. Typical load-CMOD curve for the maximum aggregate set samples

Table 2. Young's Modulus and Fracture Toughness (K_Ic)

Images were acquired with a confocal laser-scanning microscope with a 2.5X lense at a magnification of 20 to produce a field size of approximately 3.5 mm ´ 3.5 mm. The z slice thickness was 18 mm. Ten images were taken for each specimen in a random grid pattern across the surface. Contrast and brightness were adjusted to maximize the brightness range of 0 to 255. The digital images are 256 ´ 512 pixels with the top half containing the topographic map and the lower half containing the "through-focus" image which appears as a focused image of the surface. All images were filtered with a median filter to reduce noise. This filter alters the image by replacing each pixel with the median z value from the original image of the pixel and 8 surrounding pixels.

Images were acquired with a CCD video camera and NIH Image software (developed at the U.S. National Institutes of Health and available on the Internet at http://rsb.info.nih.gov/nih-image/). The specimens were coated with a flat white opaque paint to eliminate color variations within the paste and aggregated. The specimens were submerged in a purple water solution to cover the peak elevation. The surface was captured by averaging 8 frames, and 8 sections approximately 3 mm square were saved as image files. The gray scale values were calibrated to the surface elevations.

Image Analysis - Roughness Number

A common parameter to describe the geometry of a surface is the roughness number (RN). This value quantifies the relation of the measured surface area to the nominal area if the surface was planar. For example, the ratio of the area of a flat surface to the nominal area would be a RN value of 1.0. In order to determine the surface area from the topographic maps, triangles are constructed between pixels, and the area is summed over all the triangles [1]. Roughness values are related to the scale at which the surface is investigated. The roughness values from the confocal and video density techniques are presented in Table 3.

Table 3. Comparison of Roughness Number

Geometrical features that are similar at different scales of resolution is the basis of fractal geometry. A fractal dimension (D) is a quantity that describes the ruggedness of a system between Euclidean dimensions. Surfaces have a Euclidean dimension of 2. However, fracture surfaces are irregular and not easily described by Euclidean geometry. The topographical data can be analyzed by a fractal dimension measurement. This measurement takes into account the self-affine nature of the surface data which scales differently in the x-y direction and the z direction. A modified Minkowski method has been applied to ordinary Portland cement (OPC) mortars and mortars with silica fume replacement [5]. The method measures the "volume" of a surface by elevation difference within a horizontal disk area known as a structuring element [6]. The fractal dimension is determined from the log-log plot of volume divided by the disk radius computed for different disk sizes vs. the disk area. The variation of the structuring element size is limited by the confocal image size, and only extends about one decade in the log-log fractal plot.

The topological surface data is also useful for examining the effect of the tortuosity of the crack on the energy required to create the surface. A micromechanical model based on the reduction in the local stress intensity factor at an aggregate when the crack path is deflected or crack front is bowed by an inclusion in a matrix can predict the increase in toughness from the matrix toughness for a planar crack [3].

The fracture mechanics model applied to the confocal microscope image data calculates an average strain energy release rate from the tilted and twisted portions of the crack front. The crack front is deflected by inclusions out of plane by a tilt angle, q, and the crack projection is bowed around the inclusions by a twist angle, f, as shown in Figure 4. The tilted crack has Mode I (opening) and Mode II (sliding) contributions to the local stress intensity; while the twisted crack has Mode I and Mode III (tearing) contributions.

Figure 4. Crack front deflection: (a) tilt, and (b) twist

The strain energy release rate, G, and the local stress intensity factors, k^t₁ and k^t₂, for a tilted segment of crack are determined by

where E and n are the Young's modulus and Poisson's ratio of the material, respectively. The strain energy release rate and the local stress intensity factors, k^T₁ and k^T₂, for a twisted segment of crack are determined by

where the angular functions are determined by resolving the normal and shear stresses of the tilted crack onto the twist plane.

The local crack path angles were determined for each segment from the difference in z elevations and horizontal distance between adjacent pixels. Figure 5 shows the surface representation and the local angles.

Figure 5. Surface schematic for micromechanical model

The toughening ratio was determined by

where K_I is equal to 1.0 and EG is calculated from equations 1-4. The model predicts an increase in the toughness from the undeflected matrix for the composite. Figure 6 shows the relationship between the mortar specimens with single sieve aggregate distributions, those with the same gradation but different maximum aggregate and the cement paste specimen. The addition of the aggregate in the matrix results in an increase in the matrix toughness which has also been reported for fiber reinforced materials [7]. The difference between the undeflected crack toughness of cement paste to that of the mortars can be explained by the presence of other mechanisms besides crack deflection. Aggregate interlock, microcracking under the exposed surfaces, and the effect of elasticity mismatch between aggregate and matrix in the interfacial zone are mechanisms that are not accounted for by the model.

Figure 6. Toughness comparison

The roughness values of the specimen set were also compared with the ratio of the toughness of the composite material to that of the undeflected toughness determined by the model as shown in Figure 7.

Figure 7. Relation of roughness to toughness

The relationship for a linear-elastic material where all the energy in fracture goes into creating the surfaces is described by eq. 6 and shown in Figure 7 [8]:

The cement paste exhibited the lowest RN values and more closely behaved as a linear-elastic material. The mortars exhibited higher RN values, and showed greater deviation from eq. 6 at the highest RN values. This result suggests that greater roughness in the crack path implies higher toughening through crack deflection, but also indicates increasing significance of other energy absorbing mechanisms.

The toughness ratio can also be related to the fractal dimension by converting the fractal equation for the area to a roughness number and approximating log(RN) by a linear function, as described in eq. 7 [8]:

where r_c is a constant based on the fractal measuring scale, and D^* is the fractal dimension increment equal to the difference between the Euclidean dimension and the fractal dimension (D^*=D-2). Assuming that D^* computed by the modified Minkowski method reflects the fractal behavior of RN (although they are measured by different construction methods), we can consider how the toughness ratio relates to fractal dimension in this study. Figure 8 shows this relationship for the mortars and the cement paste, and indicates a clear difference between the behavior of control pastes and mortars. The constructed lines in Figure 8 are relationships proposed by Xin et al.[8] for a linear elastic material in eq. 8. This underscores a key difference between the two parameters, RN and D. Fractal dimension is not just another way to describe roughness, but rather describes the manner in which RN scales with resolution of measurement. RN is a parameter that describes surface area at a given resolution; D tells us how RN changes as resolution changes. The mortars tend to share a common D, but the D for the cement paste samples does not fit into the cluster of mortar data points. The result emphasizes a fundamental difference between the fracture of pastes and mortars. The presence of sand in mortar tends to distribute stress and permit the crack to become dominated by grain shape as the crack path weaves its way around the inclusions. Cracks in pastes with no inclusions (at the size scale of millimeters) can propagate more freely before arrest.

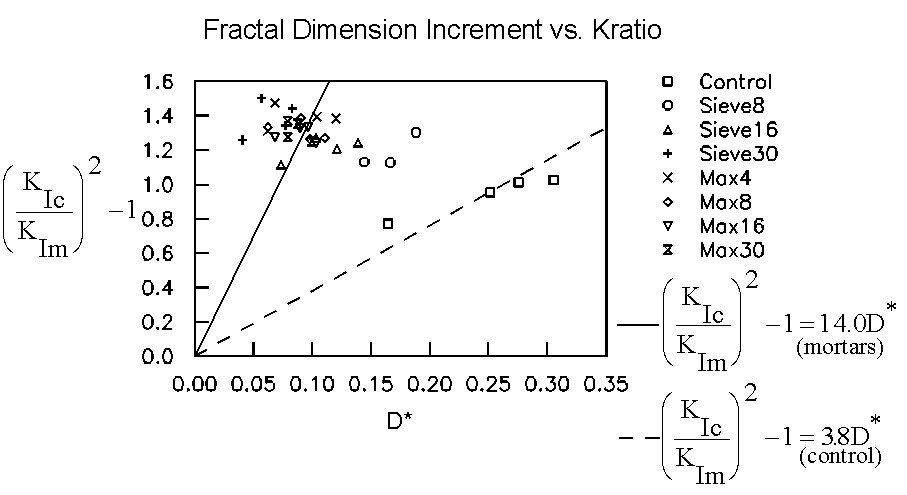
Figure 8. Relation of fractal dimension to toughness

From the investigation of fracture surface geometry, the mechanical behavior of mortars can be better understood. The mechanisms involved in the process such as crack deflection, can be recorded through optical techniques of confocal microscopy and video density in the form of topographic maps. Through image analysis, parameter such as roughness and fractal dimension can be obtained and compared to the material toughness. Also, the surface information can be input into a micromechanical model to determine the fracture toughness increase due to the inclusion of aggregate in a matrix. The model was applied to topology data for cement paste and mortar samples of differing aggregate sizes and maximum aggregate sizes. The model showed that crack deflection is a major source of toughening, but did not fully predict the toughness of paste and mortar. This is an indication that other mechanisms contribute to the energy of fracture. The relation of the toughening ratio to the roughness value illustrates the difference in material behavior when aggregate is added to cement paste and shows how the fracture behavior of cement-based materials deviates more from linear elasticity as fracture surface roughness increases.

This research was supported by the NSF Center for Advanced Cement-Based Materials (NSF Grant No. DMR 88808423-01).

The Ottowa sand was generously donated by U.S. Silica, Ottowa, IL.

1. Lange, D. A., Jennings, H. M., Shah, S. P. (1993). "Analysis of surface roughness using confocal microscopy", Journal of Materials Science, 28, 3879-3884.

2. Issa, M. A., Hammad, A. M. and Chudnovsky, A. (1993). "Correlation between crack tortuosity and fracture toughness in cementitious material", International Journal of Fracture, 60, 97-105,.

3. Faber, K. T. and Evans, A. G. (1983). "Crack Deflection Processes -I. Theory", Acta metall., 31(4), 565-576.

4. RILEM Draft Recommendations, TC89-FMT Fracture Mechanics of Concrete - Test Methods (1990). "Determination of fracture parameters (KS_Ic and CTOD_c) of plain concrete using three-point bend tests", Materials and Structures, 23, 457-460.

5. Abell, A. B. and Lange, D. A. (1994). "Image-Based Characterization of Fracture Surface Roughness", Mat. Res. Soc. Symp. Proc., 370, 107-113.

6. Russ, J.C. (1994). Fractal Surfaces, Plenum Press, New York, N.Y.

7.Lange, D.A., Sun, G.K., Bloom, R. (1994). "Fracture of Microfiber reinforced DSP Materials",Cement Transactions

8. Xin, Y., Hsia, K .J., Lange, D. A. (1995). "Quantitative Characterization of the Fracture Surface of Si Single Crystals by Confocal Microscopy", Journal of the American Ceramic Society, 78, 3201-3208.(Amer. Ceramic Society), 40, 239-246.

Return to Home